Here we use standard probability equations to produce curves shown.

Our approach follows a line of classical reasoning like this: Assume stochastics that no two physical (i.e., actual) coherent quantum comtexts' chaos~equilibria interrelationshipings are evolutionarily comsistent out of a group of 'n' energy~wellings in 't' total possible energy~wellings, and that no two physical (i.e., actual) decoherent quantum comtexts' chaos~equilibria interrelationshipings are evolutionarily comsistent out of a group of 'n' energy~wellings in 't' total possible energy~wellings is, S(n,t)=t!/((t-n)! * tn). Then we assume stochastics that two or more physical quantum comtexts are more equilibrial and less chaotic out of a group of 'n' coherent energy~wellings, and that two or more physical quantum comtexts are more chaotic and less equilibrial out of a group of 'n' decoherent energy~wellings is, P(n,t) = 1 - S(n,t). We approximated our calculation of S(n,t) using (1-n/(2*d))n-1 Classical Problematics (need wingdings font for our fickle quantum fingers :): Analyticity (determinism, induction, cause-effect, homogeneous time or independent variable as change, axiom of independence (a strict derivation of Aristotle's 3rd syllogistic 'law,' claiming excluded-middle 'independence' of classical objects), et al.) - 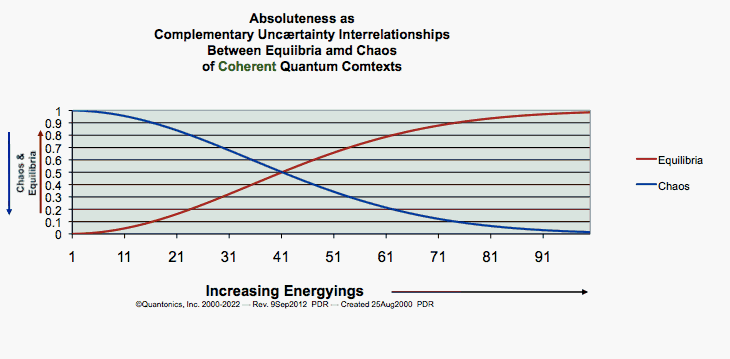As you can see in our graphs above and our classical equations, a classical assumption of monism as continuous and unitemporal (from a purely classical-ideal "reality is stoppable" view...atemporal) analyticity is inherent. Our classical equation is continuous. But is reality analytic? N¤! Reality is quantal, as graph just above illustrates. Here is an exemplar: Do our continuous, analytic stochastic graphs of our probability~plausibility~likelihood functions for classical equilibria and chaos depict quantal reality? N¤! However, we benefit from making these classical comparisons, since they show us chaoequil interrelationshipings vary with coherent~decoherent quantum~comtextings. If this makes you thinkq of PBings(cohera,decohera) and PBings(equilibria,chaos), Doug has achieved a partial quantum~affectation. Also, these two graphics illustrate a great quantum tell: quantum~reality is complementary. Complementarity appears ubiquitous and perpetual in all quantum~reality at all scales of quantum~reality. Chaos complements equilibrium and vice versa. Coherence complements decoherence and vice versa. Latter is an essential of quantum~evolutionary~creation processings as exhibited in both QED and QCD, and other omnisciplines like Bohm's Hologramic~Quantum~Theory, Bergson's Creation Philosophy, Quantonics, Qabala, and its associated cosmic energy language: Autiot, etc. Doug - 10Sep2012. Localability, isolability/individuicity, separability, and reducibility (lisr) - A classical assumption of lisr is inherent in classical reasoning. Each classical context is lisr. Each classical context adheres Aristotelian syllogisms, especially objective, excluded-middles among all classical contexts. But is reality lisr? N¤! Reality is quantum c¤hesive. Do our continuous, analytic graphs of our probability functions for classical equilibria and chaos depict quantum c¤hesive reality? Yæs, partially! They show quanton(chaos,equilibrium) as quantum~complementary and thus, to some extent, quantum~coherent due an Quantonics assumption of quantum~middle~inclusion. Ditto quanton(coherence,decoherence). Trouble is, those analytic graphs do it using classical maths' canonic Aristotelian sillygisms! Ideal mathematical integer constancy - A classical assumption that an ideal logical/physical constant, one (1), exists. A classical assumption that ideal logical/physical manipulations of one (1) exist: 1/1 iso 1 1*1 iso 1 1+1 iso 2 (inference of induction and counting) 1-1 iso 0 (inherent definition of zero) 1 = 1 iso classical ideal identity d1/dt = 0 (1st derivative of a 'constant' is zero; assumes homogeneous time) (1-1)/(1-1) iso undefined (Isn't this amazing?) But does reality generate or manifest physical integer constants? N¤! All quantum n¤mbærs are umcærtain! N¤ tw¤ quantum n¤mbærs are identical! Too, they are perpetually and ubiquitously evolving. In general it is n¤t p¤ssible f¤r tw¤ quantum n¤mbærs t¤ be physically and thus l¤gically identical. Do our continuous, analytic graphs of our probability functions for classical equilibria and chaos depict quantum n¤mbær umcærtainty? N¤! (I.e., classical use of '1-P.') Quantum~n¤mbærs are dynamic, evolving processings. For example, see Doug's Quantum~Hamiltonian. See Doug's list of Suggested Requirements for A New Quantum~Mathematics. So, reader, you can see how classical concepts, especially classical mathematical sillygistic concepts, impede any real and natural understanding of quantum~reality. N¤ mathematician ever shows '1' as a quantum umcærtain comcept. (Using n¤vel memes presented here we can d¤ that.) F¤r ¤ur purp¤ses here, let's assume that ¤ur least values f¤r equilibria and chaos in ¤ur n¤nclassical, quantum~reality are individual quanta. Our curves d¤ n¤t sh¤w that; h¤wever, we can imagine their classical zero asymptotes as reality's minimum Planck quantum, i.e., quantum_2•quantum_•h.